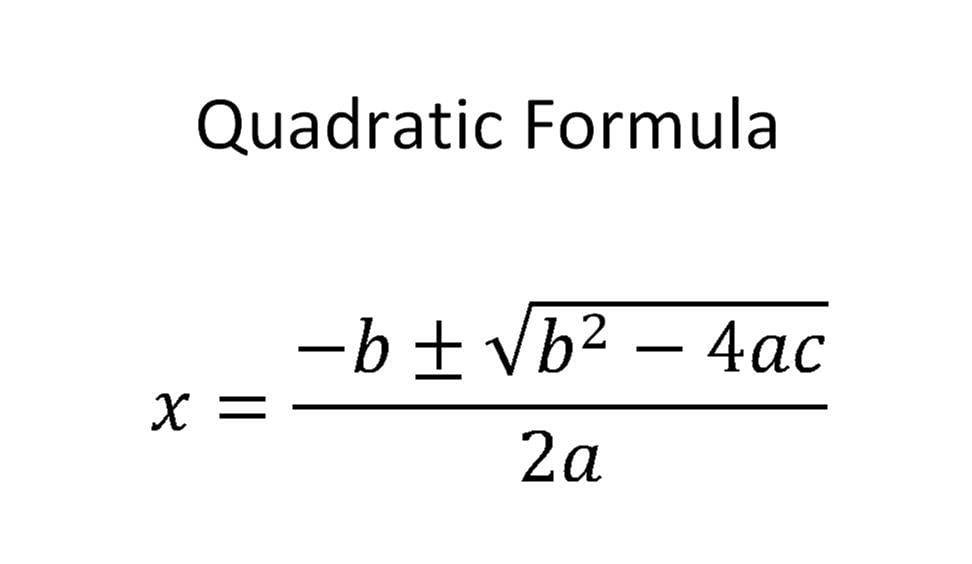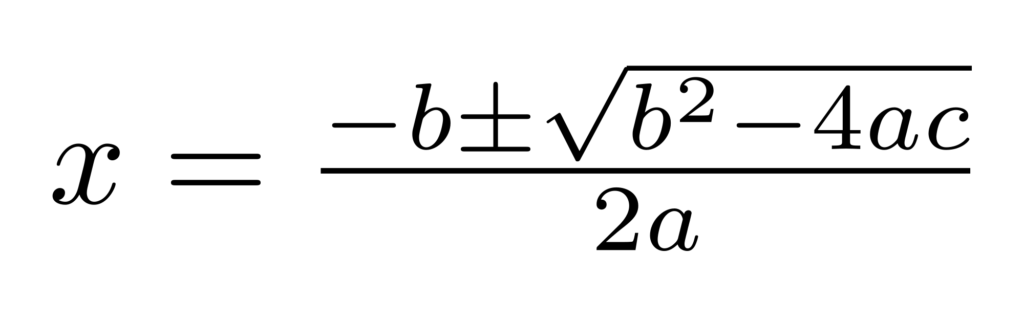3 minute read
Even mathematicians admit that the quadratic formula can look messy and overwhelming.
Try not to allow the multitude of letters, numbers and signs distract you from the importance of this very critical equation.
But what is the quadratic equation used for in real life?
Well, not only is it highly useful to you as a student studying math topics, but it has relevance in other subject areas, including physics and engineering.
Rather than see the quadratic equation as an overwhelming problem, let’s break it down step by step.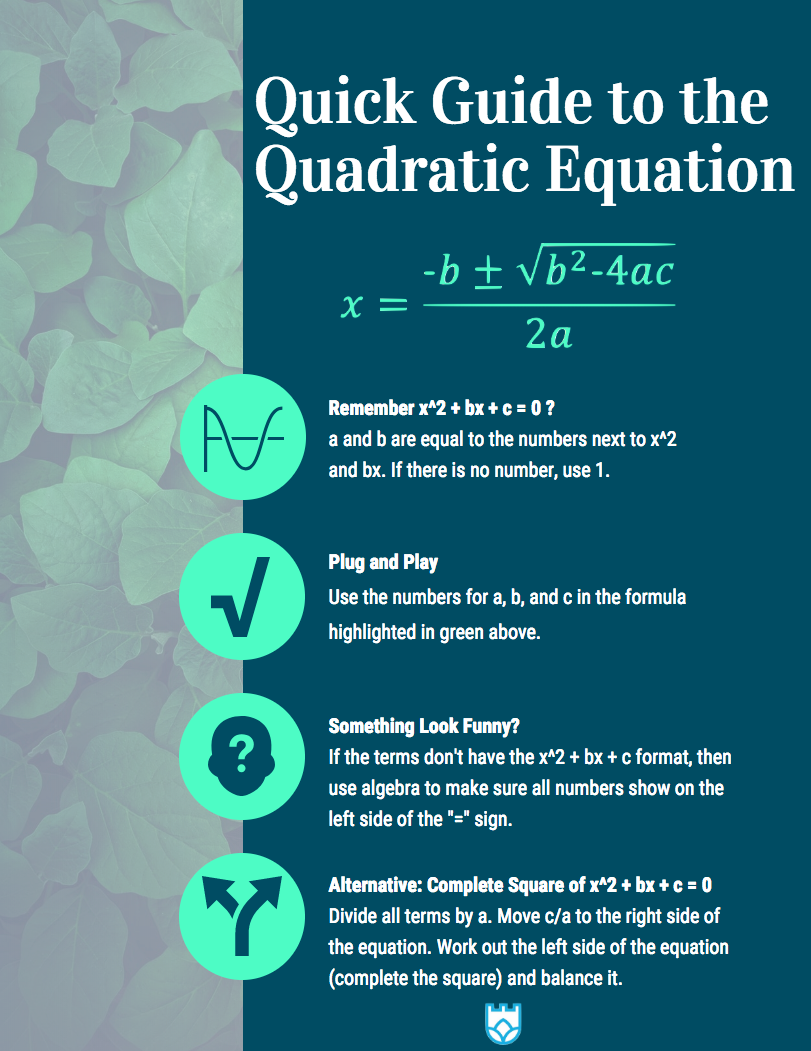
What the Quadratic Formula Means
When you see c, you are looking at a quadratic.
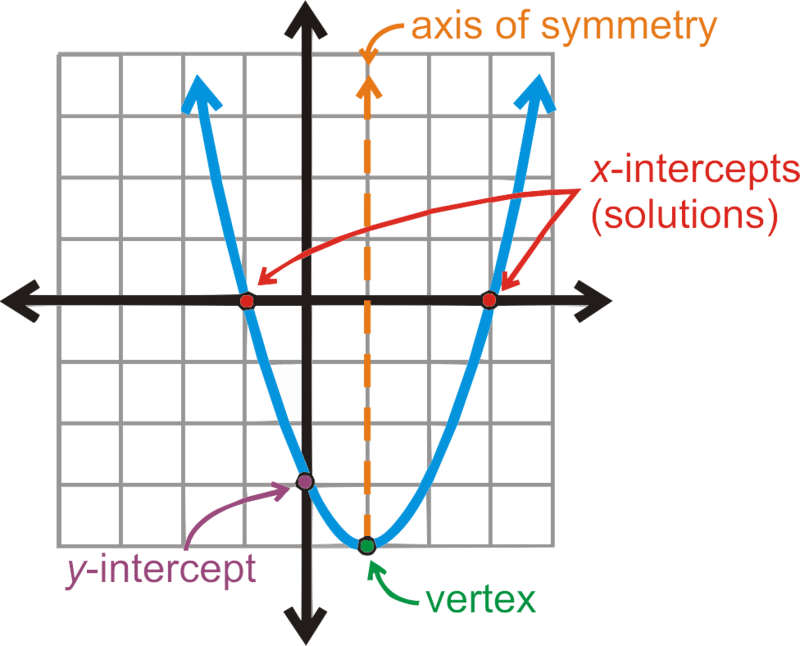
The “x” corresponds with a specific line on a graph, usually the horizontal line.
The vertical line of the graph, or “y” is understood as the “0”. The “a”, “b”, and “c” elements are simply place marks for numbers.
A quadratic always gives you two answers for “x” so you can quickly see where they fall on the graph when “y” is zero.
An Easy Example for You to Work Through
Some quadratics are very clean; you may even be able to work them out in your head.
Here are some quadratic formula examples.
x^2 – 3x – 4= 0 works out to (x – 4) x (x + 1) = 0. This means your two “x” answers are “4” and “-1”.
Knowing this, you could plot those places on a graph and connect them with a curving line called a parabola.
The high point, or vertex, of the parabola will correspond with the “x” line at -b/2a.
For our sample above, with “b” as “-3” and “a” as “-4”, the result is 3/-8 or, in decimals, “-.375”.
We can plug “-3/8” into the original equation and find that y = (-3/8)^2 + 9/8 – 4.The answer comes to “-2.75”.
Thus, we know the vertex of our quadratic equation’s corresponding parabola is at coordinates “-.375, -2.75.”
Khan Academy does a great job of explaining how to work through basic equations.
What Happens When Quadratics Are Complicated?
Our sample quadratics and corresponding parabola was relatively uncomplicated.
However, when you have quadratics that don’t come together easily, you must use the quadratic equation.
The quadratic formula looks quite complex but is just another way of looking at ax^2 + bx + c = 0.
Consequently, it involves solving a top half, -b +/- the square root of b^2 – 4ac, and then dividing the top half by 2a.
This will give you the answer to “x” just as well as ax^2 + bx + c = 0.
We’ll look at the problem above to make sure it works:
Our quadratic: x^2 – 3x – 4= 0
“-b” equals “3”
“b^2” equals “9”
“-4ac” equals “16”
“2a” equals “2”
Consequently, our answer is x = 3 +/- the square root of 25 divided by 2, or x = 4 and x = -1. These are the exact solutions we found at the beginning of our journey.
Give Yourself Time to Understand the Quadratic Formula Steps
For most people, mastering quadratics is a process that requires a lot of time and energy.
Plan to work out problems on your own time, and you’ll start to get faster and more accurate, especially when you decide to start getting homework help with equations. In the meantime, I recommend you start with this guide on how to solve linear equations.
Then, you can begin to apply the quadratic formula to plenty of real-life scenarios, from the height of a baseball tossed into the air at a particular velocity, to the time it takes a missile launched at a certain rate to hit its target.
Updated February 16, 2019 with an infographic